Seminar : Virginie Ehrlacher

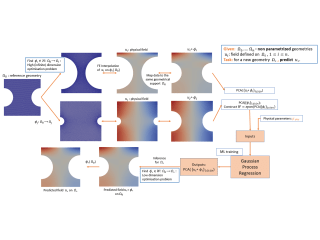
The aim of this presentation is to present the first recent results obtained on a new morphing method for constructing reduced models. The aim of this work in fine is to accelerate the simulation time of mechanical problems where the geometry of the domain occupied by the solid of interest is variable, but cannot be described by means of a small number of parameters. The numerical method is based on several steps. First, assuming we have a database of geometries and fields of interest (displacements, stresses), we try to calculate a diffeomorphism between each geometry in the database and a reference geometry. These diffeomorphisms are then optimised so that the fields of interest deformed by these diffeomorphisms can be best compressed using a POD decomposition. These two steps constitute the "offline" stage of the method. We will then present an efficient online method for the rapid calculation of fields of interest linked to a new geometry, based on the efficient calculation of an optimised diffeomorphism between the new geometry and the reference geometry. We will illustrate the behaviour of the method on several interesting test cases. This work is the result of a collaboration with Felipe Bordeu, Fabien Casenave Alexandre Ern and Abbas Kabalan.